我們知道調和級數 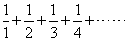 發散 發散 但當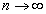 時, 時, 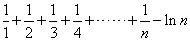 收斂到一個 0到1之間的數,我們定義為 收斂到一個 0到1之間的數,我們定義為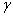 (讀gamma),也就是說 (讀gamma),也就是說

證明: 令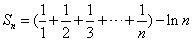 ,則 ,則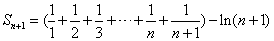 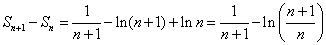
若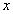 為小於 為小於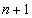 的實數,也就是說 的實數,也就是說 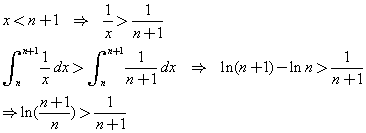
因此可以得知 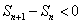 ,也就是說 ,也就是說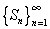 為遞減數列 為遞減數列
再假設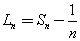 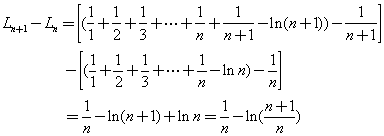
若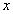 為大於 為大於 的實數,也就是說 的實數,也就是說 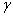 的近似值 的近似值
n值 | 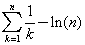
| 10 | 0.626383161 | 100 | 0.582207332 | 1000 | 0.577715582 | 10000 | 0.577265664 | 100000 | 0.577265664 | 1000000 | 0.57721616 |
> evalf(sum(1/i,i=1..1000000)-ln(1000000)); 0.57721616 |


